The Fibonacci Numbers

The Fibonacci Numbers were first studied by Leonard of Pisa (1175 – 1250 A.D.).  Leonardo, better known as Fibonacci, made numerous contributions to mathematics including the introduction of the decimal number system to Europe. However, he is best remembered for the numeric series which bears his name the Fibonacci Series. This series of numbers has since been considered a marvel to mathematicians and laymen alike. The Fibonacci Series is quite simple to construct per the following rule:

In simple English, this equation states that a number N in the series is equal to the sum of the previous two numbers in the series. This leads to the following example of the first 10 integers in the Fibonacci Series:

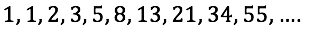
Among many other interesting features of the Fibonacci Series is one that links these marvellous numbers to my Fibonacci based compositions. If you divide any number in the series by its predecessor you get an approximation of the Golden Number PHI (1.617…):

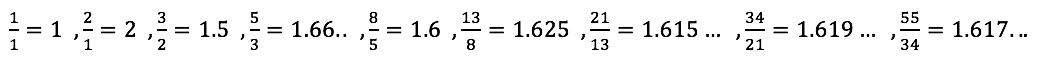
Notice how larger numbers in the series result in closer approximations to PHI. Manifestations of this number, PHI, can be found all over in nature and mathematics. And research has shown that PHI plays a role in what humans visually perceive to be beautiful.

In the Fibonacci Series my aim is to create purely abstract images based on this elegant and beautiful mathematical concept. The goal, of course, is that it will result in a visually appealing composition. The compositions consist of interlocking layers of stripes that are in Fibonacci proportions to one another. Starting at corners with square 1, the square 2, square 3 and so forth. The series expands from each corner to the center. The result is a harmony of ratios and color that is delightful to look at. The human brain is a pattern recognition machine and finding patterns activates deep circuits in the brain. The patterns of fibonacci based compositions are familiar and captivating to the human brain.

It is my hope that you will enjoy this work and marvel in its links to mathematics. Perhaps, in closer study, it will reveal to you other interesting manifestations of PHI and the Fibonacci Series